Priestley duality and representations of recurrent dynamics

William Kalies and Robert Vandervorst

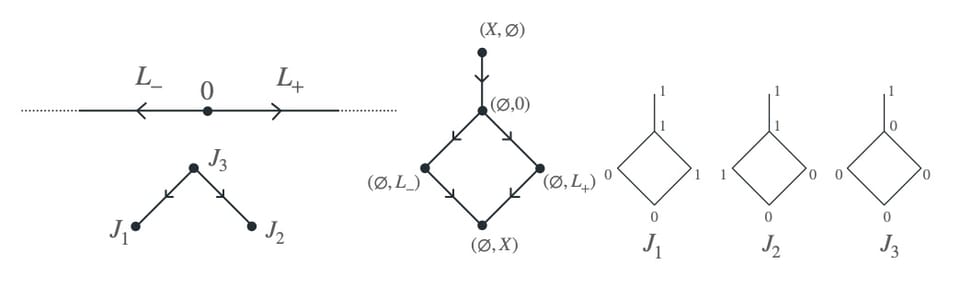
For an arbitrary dynamical system there is a strong relationship between global dynamics and the order structure of an appropriately constructed Priestley space. This connection provides an order-theoretic framework for studying global dynamics. In the classical setting, the chain recurrent set, introduced by C. Conley, is an example of an ordered Stone space or Priestley space. Priestley duality can be applied in the setting of dynamics on arbitrary topological spaces and yields a notion of Hausdorff compactification of the (chain) recurrent set.

Priestley duality and representations of recurrent dynamics

For an arbitrary dynamical system there is a strong relationship between global dynamics and the order structure of an appropriately constructed Priestley space. This connection provides an order-theoretic framework for studying global dynamics. In the classical setting, the chain recurrent set, introduced by C. Conley, is an example of an ordered Stone space or Priestley space. Priestley duality can be applied in the setting of dynamics on arbitrary topological spaces and yields a notion of Hausdorff compactification of the (chain) recurrent set.

arXiv.orgWilliam Kalies